Constant Temperature Accelerated Life Testing using the Arrhenius Relationship

When products are mainly exposed to temperature stresses in the field, Constant Temperature Accelerated Life Testing is used to simulate product life. Products can be tested at temperatures above their normal use temperature during Constant Temperature Accelerated Life Testing in order to accelerate aging. Defects or failure modes that would show up after many years in the field at normal use temperatures can be detected in short times in an Accelerated Life Test. In Constant Temperature Accelerated Life Testing, the typical failure mode is dependent on migration/diffusion or chemical reactions. These types of failures are typically found in electronic components but can also occur in other types of products or materials such as adhesives, batteries, etc. The Arrhenius Equation relates reaction rates to temperature and is used to correlate time in the field at normal use temperature to a Constant Temperature Accelerated Life Test. It should be noted that constant temperature testing will not precipitate failure modes due to thermal cycling. Temperature or thermal cycle testing will be discussed in another blog article.

The Arrhenius Equation that relates reaction rates to temperature is:

 

 

 

 

 

 

The Acceleration Factor (AF) can be obtained by the ratio of the reaction rates at two different temperatures and is given by the following equation:

 

 

 

 

 

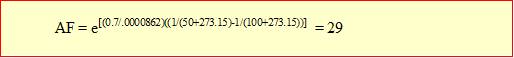
As an example, consider a product with a normal operating temperature of 50 °C. Testing the product at 100 °C will result in the following acceleration factor, assuming an activation energy of 0.7 eV:

 

 

 

Testing the product for 1,000 hours (≈ 6 weeks) at 100 °C is therefore equivalent to 29,000 hours or 3.3 years of life at the normal operating temperature of 50 °C. Increasing the test temperature will increase the acceleration. For example, if the same sample could be tested at 130 °C, the acceleration factor (AF) would increase to 146 so that the same 1,000 hours of testing would now be equivalent to 16.7 years of life at the normal use temperature. However, care must be taken such that the test temperature is not higher than what the product can withstand. Using too high of a test temperature may result in unrealistic failures that would not occur during normal product operating temperatures. It is therefore always important to evaluate failures obtained during accelerated temperature testing to determine if they are the same type of failure that would be expected to occur at normal operating temperatures.

Using the correct value for the activation energy is also important as a small change in the activation energy will have an effect on the acceleration factor. Activation energy values for various processes have been reported and can be found in the literature or the Internet. It is also possible to experimentally determine the activation energy by performing tests at multiple temperatures and plotting the results.

What sets Delserro Engineering Solutions, Inc. (DES) apart from other labs is our knowledge on how to relate test time to time in the field. So if you do not know what test conditions that you should use, what specification to choose, or how to correlate your test to field life, then we will help you because we are reliability testing experts!